Draw Pentagon Inside a Circle

Shape with v sides

This article is about the geometric figure. For the headquarters of the United States Department of Defense force, encounter The Pentagon. For other uses, encounter Pentagon (disambiguation).

Pentagon
An equilateral pentagon, i.due east. a pentagon whose five sides all take the same length
Edges and vertices	5
Internal angle (degrees)	108° (if equiangular, including regular)

In geometry, a pentagon (from the Greek πέντε pente meaning v and γωνία gonia significant angle ^[ane]) is whatever five-sided polygon or 5-gon. The sum of the internal angles in a uncomplicated pentagon is 540°.

A pentagon may exist simple or self-intersecting. A self-intersecting regular pentagon (or star pentagon) is called a pentagram.

Regular pentagons [edit]

Regular pentagon
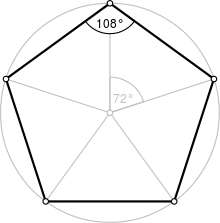A regular pentagon
Type	Regular polygon
Edges and vertices	5
Schläfli symbol	{5}
Coxeter–Dynkin diagrams
Symmetry grouping	Dihedral (D5), gild two×v
Internal bending (degrees)	108°
Properties	Convex, circadian, equilateral, isogonal, isotoxal

A regular pentagon has Schläfli symbol {5} and interior angles of 108°.

A regular pentagon has v lines of reflectional symmetry, and rotational symmetry of gild 5 (through 72°, 144°, 216° and 288°). The diagonals of a convex regular pentagon are in the golden ratio to its sides. Given its side length ${\displaystyle t,}$ its height ${\displaystyle H}$ (distance from ane side to the opposite vertex), width ${\displaystyle West}$ (distance between ii farthest separated points, which equals the diagonal length ${\displaystyle D}$ ) and circumradius ${\displaystyle R}$ are given past:

${\displaystyle {\begin{aligned}H&={\frac {\sqrt {5+ii{\sqrt {5}}}}{2}}~t\approx 1.539~t,\\West=D&={\frac {1+{\sqrt {five}}}{ii}}~t\approx ane.618~t,\\Due west&={\sqrt {2-{\frac {2}{\sqrt {5}}}}}\cdot H\approx 1.051~H,\\R&={\sqrt {\frac {v+{\sqrt {5}}}{10}}}\approx 0.8507~t,\\D&=R\ {\sqrt {\frac {v+{\sqrt {five}}}{2}}}=2R\cos 18^{\circ }=2R\cos {\frac {\pi }{10}}\approx 1.902~R.\end{aligned}}}$

The surface area of a convex regular pentagon with side length ${\displaystyle t}$ is given past

${\displaystyle {\begin{aligned}A&={\frac {t^{2}{\sqrt {25+ten{\sqrt {v}}}}}{4}}={\frac {5t^{2}\tan 54^{\circ }}{four}}\\&={\frac {{\sqrt {5(5+2{\sqrt {5}})}}\;t^{2}}{iv}}\approx one.720~t^{2}.\end{aligned}}}$

If the circumradius ${\displaystyle R}$ of a regular pentagon is given, its border length ${\displaystyle t}$ is found by the expression

${\displaystyle t=R\ {\sqrt {\frac {five-{\sqrt {5}}}{2}}}=2R\sin 36^{\circ }=2R\sin {\frac {\pi }{5}}\approx 1.176~R,}$

and its expanse is

${\displaystyle A={\frac {5R^{2}}{4}}{\sqrt {\frac {5+{\sqrt {5}}}{2}}};}$

since the area of the confining circle is ${\displaystyle \pi R^{ii},}$ the regular pentagon fills approximately 0.7568 of its circumscribed circumvolve.

Derivation of the expanse formula [edit]

The surface area of whatsoever regular polygon is:

${\displaystyle A={\frac {ane}{two}}Pr}$

where P is the perimeter of the polygon, and r is the inradius (equivalently the apothem). Substituting the regular pentagon's values for P and r gives the formula

${\displaystyle A={\frac {1}{2}}\cdot 5t\cdot {\frac {t\tan {\mathord {\left({\frac {3\pi }{ten}}\right)}}}{ii}}={\frac {5t^{2}\tan {\mathord {\left({\frac {3\pi }{x}}\correct)}}}{iv}}}$

with side length t.

Inradius [edit]

Like to every regular convex polygon, the regular convex pentagon has an inscribed circle. The apothem, which is the radius r of the inscribed circumvolve, of a regular pentagon is related to the side length t by

${\displaystyle r={\frac {t}{2\tan {\mathord {\left({\frac {\pi }{v}}\right)}}}}={\frac {t}{2{\sqrt {v-{\sqrt {twenty}}}}}}\approx 0.6882\cdot t.}$

Chords from the circumscribed circle to the vertices [edit]

Like every regular convex polygon, the regular convex pentagon has a circumscribed circle. For a regular pentagon with successive vertices A, B, C, D, Due east, if P is whatsoever point on the circumcircle between points B and C, so PA + PD = Lead + PC + PE.

Indicate in plane [edit]

For an arbitrary point in the aeroplane of a regular pentagon with circumradius ${\displaystyle R}$ , whose distances to the centroid of the regular pentagon and its five vertices are ${\displaystyle L}$ and ${\displaystyle d_{i}}$ respectively, we take ^[2]

${\displaystyle {\begin{aligned}\textstyle \sum _{i=1}^{5}d_{i}^{2}&=v\left(R^{2}+L^{two}\right),\\\textstyle \sum _{i=1}^{5}d_{i}^{4}&=v\left(\left(R^{2}+50^{ii}\right)^{2}+2R^{2}L^{2}\right),\\\textstyle \sum _{i=1}^{5}d_{i}^{6}&=5\left(\left(R^{2}+50^{ii}\right)^{three}+6R^{2}Fifty^{2}\left(R^{2}+L^{2}\right)\correct),\\\textstyle \sum _{i=i}^{five}d_{i}^{8}&=5\left(\left(R^{2}+L^{ii}\right)^{4}+12R^{2}L^{2}\left(R^{two}+L^{2}\right)^{2}+6R^{4}L^{4}\correct).\end{aligned}}}$

If ${\displaystyle d_{i}}$ are the distances from the vertices of a regular pentagon to whatsoever point on its circumcircle, and then ^[ii]

${\displaystyle 3\left(\textstyle \sum _{i=1}^{5}d_{i}^{2}\right)^{two}=10\textstyle \sum _{i=1}^{v}d_{i}^{4}.}$

Geometrical constructions [edit]

The regular pentagon is constructible with compass and straightedge, equally 5 is a Fermat prime. A diversity of methods are known for constructing a regular pentagon. Some are discussed below.

Richmond's method [edit]

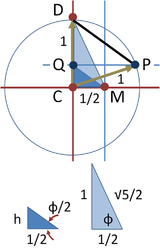
1 method to construct a regular pentagon in a given circle is described by Richmond^[3] and further discussed in Cromwell'south Polyhedra.^[4]

The top panel shows the construction used in Richmond's method to create the side of the inscribed pentagon. The circumvolve defining the pentagon has unit radius. Its center is located at signal C and a midpoint Thousand is marked halfway along its radius. This point is joined to the periphery vertically above the middle at bespeak D. Angle CMD is bisected, and the bisector intersects the vertical axis at point Q. A horizontal line through Q intersects the circumvolve at point P, and chord PD is the required side of the inscribed pentagon.

To decide the length of this side, the ii right triangles DCM and QCM are depicted below the circle. Using Pythagoras' theorem and two sides, the hypotenuse of the larger triangle is found equally ${\displaystyle \scriptstyle {\sqrt {v}}/2}$ . Side h of the smaller triangle so is establish using the half-angle formula:

${\displaystyle \tan(\phi /two)={\frac {ane-\cos(\phi )}{\sin(\phi )}}\ ,}$

where cosine and sine of ϕ are known from the larger triangle. The result is:

${\displaystyle h={\frac {{\sqrt {5}}-1}{iv}}\ .}$

If DP is truly the side of a regular pentagon, ${\displaystyle chiliad\angle \mathrm {CDP} =54^{\circ }}$ , and then DP = 2 cos(54°), QD = DP cos(54°) = 2cosⁱⁱ(54°), and CQ = i − 2cos²(54°), which equals −cos(108°) past the cosine double bending formula. This is the cosine of 72°, which equals ${\displaystyle \left({\sqrt {5}}-1\right)/4}$ as desired.

Carlyle circles [edit]

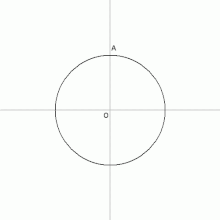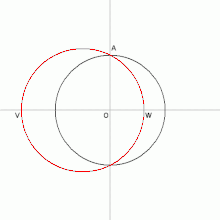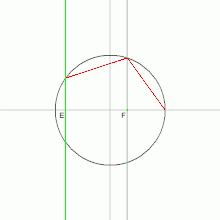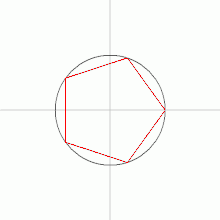
Method using Carlyle circles

The Carlyle circle was invented equally a geometric method to discover the roots of a quadratic equation.^[v] This methodology leads to a procedure for amalgam a regular pentagon. The steps are as follows:^[6]

1. Draw a circle in which to inscribe the pentagon and mark the eye signal O.
2. Draw a horizontal line through the eye of the circumvolve. Mark the left intersection with the circle every bit point B.
3. Construct a vertical line through the center. Mark one intersection with the circle as point A.
4. Construct the point M every bit the midpoint of O and B.
5. Draw a circle centered at 1000 through the point A. Marking its intersection with the horizontal line (within the original circumvolve) as the point W and its intersection exterior the circle as the indicate V.
6. Draw a circumvolve of radius OA and center Westward. It intersects the original circle at two of the vertices of the pentagon.
7. Draw a circle of radius OA and center V. It intersects the original circle at 2 of the vertices of the pentagon.
8. The 5th vertex is the rightmost intersection of the horizontal line with the original circle.

Steps 6–8 are equivalent to the following version, shown in the animation:

6a. Construct point F as the midpoint of O and Westward.

7a. Construct a vertical line through F. It intersects the original circumvolve at ii of the vertices of the pentagon. The 3rd vertex is the rightmost intersection of the horizontal line with the original circle.

8a. Construct the other two vertices using the compass and the length of the vertex establish in footstep 7a.

Euclid's method [edit]

Euclid's method for pentagon at a given circumvolve, using of the gilded triangle, animation 1 min 39 s

A regular pentagon is constructible using a compass and straightedge, either past inscribing one in a given circle or constructing one on a given border. This process was described past Euclid in his Elements circa 300 BC.^{[7] [eight]}

Physical construction methods [edit]

Overhand knot of a paper strip

• A regular pentagon may exist created from just a strip of paper by tying an overhand knot into the strip and carefully flattening the knot by pulling the ends of the newspaper strip. Folding one of the ends back over the pentagon will reveal a pentagram when backlit.
• Construct a regular hexagon on stiff paper or bill of fare. Crease along the 3 diameters between opposite vertices. Cut from i vertex to the center to make an equilateral triangular flap. Fix this flap underneath its neighbor to make a pentagonal pyramid. The base of operations of the pyramid is a regular pentagon.

Symmetry [edit]

Symmetries of a regular pentagon. Vertices are colored past their symmetry positions. Blue mirror lines are drawn through vertices and edges. Gyration orders are given in the center.

The regular pentagon has Dih₅ symmetry, order 10. Since 5 is a prime number there is one subgroup with dihedral symmetry: Dih₁, and 2 cyclic group symmetries: Z_v, and Z₁.

These iv symmetries tin can exist seen in 4 singled-out symmetries on the pentagon. John Conway labels these by a alphabetic character and group order.^[nine] Full symmetry of the regular course is r10 and no symmetry is labeled a1. The dihedral symmetries are divided depending on whether they pass through vertices (d for diagonal) or edges (p for perpendiculars), and i when reflection lines path through both edges and vertices. Cyclic symmetries in the middle column are labeled every bit g for their cardinal gyration orders.

Each subgroup symmetry allows one or more degrees of freedom for irregular forms. Simply the g5 subgroup has no degrees of freedom merely tin can exist seen as directed edges.

Regular pentagram [edit]

A pentagram or pentangle is a regular star pentagon. Its Schläfli symbol is {5/2}. Its sides form the diagonals of a regular convex pentagon – in this arrangement the sides of the two pentagons are in the aureate ratio.

Equilateral pentagons [edit]

Equilateral pentagon built with four equal circles disposed in a chain.

An equilateral pentagon is a polygon with five sides of equal length. Nevertheless, its five internal angles tin can take a range of sets of values, thus permitting it to form a family of pentagons. In dissimilarity, the regular pentagon is unique up to similarity, because information technology is equilateral and information technology is equiangular (its v angles are equal).

Circadian pentagons [edit]

A cyclic pentagon is one for which a circle chosen the circumcircle goes through all v vertices. The regular pentagon is an instance of a cyclic pentagon. The area of a cyclic pentagon, whether regular or not, can exist expressed as 1 quaternary the square root of one of the roots of a septic equation whose coefficients are functions of the sides of the pentagon.^{[10] [11] [12]}

There exist cyclic pentagons with rational sides and rational area; these are called Robbins pentagons. It has been proven that the diagonals of a Robbins pentagon must be either all rational or all irrational, and it is conjectured that all the diagonals must be rational.^[13]

General convex pentagons [edit]

For all convex pentagons, the sum of the squares of the diagonals is less than three times the sum of the squares of the sides.^{[fourteen] : p.75, #1854}

Pentagons in tiling [edit]

A regular pentagon cannot appear in whatsoever tiling of regular polygons. First, to testify a pentagon cannot form a regular tiling (ane in which all faces are congruent, thus requiring that all the polygons be pentagons), observe that 360° / 108° = iii one⁄3 (where 108° Is the interior angle), which is not a whole number; hence at that place exists no integer number of pentagons sharing a unmarried vertex and leaving no gaps between them. More difficult is proving a pentagon cannot be in any edge-to-edge tiling fabricated past regular polygons:

The maximum known packing density of a regular pentagon is approximately 0.921, accomplished by the double lattice packing shown. In a preprint released in 2016, Thomas Hales and Wöden Kusner announced a proof that the double lattice packing of the regular pentagon (which they call the "pentagonal ice-ray" packing, and which they trace to the work of Chinese artisans in 1900) has the optimal density amid all packings of regular pentagons in the plane.^[xv] As of 2020^[update], their proof has not yet been refereed and published.

At that place are no combinations of regular polygons with 4 or more meeting at a vertex that incorporate a pentagon. For combinations with 3, if 3 polygons meet at a vertex and 1 has an odd number of sides, the other 2 must exist congruent. The reason for this is that the polygons that bear on the edges of the pentagon must alternating around the pentagon, which is impossible considering of the pentagon's odd number of sides. For the pentagon, this results in a polygon whose angles are all (360 − 108) / ii = 126°. To find the number of sides this polygon has, the event is 360 / (180 − 126) = vi 2⁄3 , which is non a whole number. Therefore, a pentagon cannot appear in any tiling fabricated by regular polygons.

In that location are 15 classes of pentagons that tin monohedrally tile the plane. None of the pentagons have any symmetry in general, although some accept special cases with mirror symmetry.

15 monohedral pentagonal tiles

1	2	three	4	v
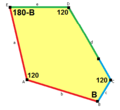6	vii	8	9	10
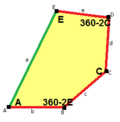eleven	12	13	14	fifteen

Pentagons in polyhedra [edit]

Ih	Th	Td	O	I	D5d
Dodecahedron	Pyritohedron	Tetartoid	Pentagonal icositetrahedron	Pentagonal hexecontahedron	Truncated trapezohedron

Pentagons in nature [edit]

Plants [edit]

• 

  Pentagonal cross-section of okra.

• 

  Starfruit is another fruit with fivefold symmetry.

Animals [edit]

• 

  Another example of echinoderm, a sea urchin endoskeleton.

• 

  An illustration of brittle stars, also echinoderms with a pentagonal shape.

Minerals [edit]

• 

  A pyritohedral crystal of pyrite. A pyritohedron has 12 identical pentagonal faces that are not constrained to be regular.

Other examples [edit]

• 

• 

Run across also [edit]

• Associahedron; A pentagon is an guild-4 associahedron
• Dodecahedron, a polyhedron whose regular form is equanimous of 12 pentagonal faces
• Gilt ratio
• List of geometric shapes
• Pentagonal numbers
• Pentagram
• Pentagram map
• Pentastar, the Chrysler logo
• Pythagoras' theorem#Like figures on the iii sides
• Trigonometric constants for a pentagon

In-line notes and references [edit]

1. ^ "pentagon, adj. and due north." OED Online. Oxford Academy Press, June 2014. Spider web. 17 August 2014.
2. ^ ^{a b} Meskhishvili, Mamuka (2020). "Cyclic Averages of Regular Polygons and Platonic Solids". Communications in Mathematics and Applications. eleven: 335–355. arXiv:2010.12340.
3. ^ Herbert W Richmond (1893). "Pentagon".
4. ^ Peter R. Cromwell (22 July 1999). Polyhedra. p. 63. ISBN0-521-66405-5.
5. ^ Eric W. Weisstein (2003). CRC curtailed encyclopedia of mathematics (2nd ed.). CRC Press. p. 329. ISBN1-58488-347-2.
6. ^ DeTemple, Duane W. (Feb 1991). "Carlyle circles and Lemoine simplicity of polygon constructions" (PDF). The American Mathematical Monthly. 98 (2): 97–108. doi:ten.2307/2323939. JSTOR 2323939. Archived from the original (PDF) on 2015-12-21.
7. ^ George Edward Martin (1998). Geometric constructions. Springer. p. six. ISBN0-387-98276-0.
8. ^ Fitzpatrick, Richard (2008). Euklid's Elements of Geometry, Volume 4, Proposition xi (PDF). Translated by Richard Fitzpatrick. p. 119. ISBN978-0-6151-7984-1.
9. ^ John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN 978-1-56881-220-5 (Chapter 20, Generalized Schaefli symbols, Types of symmetry of a polygon pp. 275-278)
10. ^ Weisstein, Eric W. "Circadian Pentagon." From MathWorld--A Wolfram Web Resource. [1]
11. ^ Robbins, D. P. (1994). "Areas of Polygons Inscribed in a Circle". Detached and Computational Geometry. 12 (ii): 223–236. doi:10.1007/bf02574377.
12. ^ Robbins, D. P. (1995). "Areas of Polygons Inscribed in a Circle". The American Mathematical Monthly. 102 (half dozen): 523–530. doi:10.2307/2974766. JSTOR 2974766.
13. ^ *Buchholz, Ralph H.; MacDougall, James A. (2008), "Cyclic polygons with rational sides and expanse", Journal of Number Theory, 128 (one): 17–48, doi:10.1016/j.jnt.2007.05.005, MR 2382768 .
14. ^ Inequalities proposed in "Crux Mathematicorum", [ii].
15. ^ Hales, Thomas; Kusner, Wöden (September 2016), Packings of regular pentagons in the plane, arXiv:1602.07220

External links [edit]

Look upwardly pentagon in Wiktionary, the free dictionary.

Wikimedia Commons has media related to Pentagons.

• Weisstein, Eric W. "Pentagon". MathWorld.
• Animated sit-in constructing an inscribed pentagon with compass and straightedge.
• How to construct a regular pentagon with but a compass and straightedge.
• How to fold a regular pentagon using only a strip of newspaper
• Definition and properties of the pentagon, with interactive animation
• Renaissance artists' approximate constructions of regular pentagons
• Pentagon. How to calculate diverse dimensions of regular pentagons.

v t eastward Cardinal convex regular and uniform polytopes in dimensions 2–10
Family	A due north	B northward	I 2(p) / D n	E 6 / East 7 / E 8 / F 4 / G 2	Hn
Regular polygon	Triangle	Square	p-gon	Hexagon	Pentagon
Uniform polyhedron	Tetrahedron	Octahedron • Cube	Demicube		Dodecahedron • Icosahedron
Uniform polychoron	Pentachoron	16-cell • Tesseract	Demitesseract	24-cell	120-cell • 600-cell
Compatible 5-polytope	v-simplex	5-orthoplex • 5-cube	5-demicube
Compatible 6-polytope	6-simplex	half-dozen-orthoplex • 6-cube	half-dozen-demicube	122 • two21
Uniform vii-polytope	seven-simplex	7-orthoplex • 7-cube	7-demicube	i32 • 231 • three21
Uniform viii-polytope	viii-simplex	eight-orthoplex • 8-cube	eight-demicube	142 • 241 • iv21
Uniform 9-polytope	nine-simplex	nine-orthoplex • ix-cube	9-demicube
Uniform 10-polytope	10-simplex	10-orthoplex • 10-cube	x-demicube
Uniform n-polytope	due north-simplex	n-orthoplex • n-cube	north-demicube	1k2 • iik1 • k21	n-pentagonal polytope
Topics: Polytope families • Regular polytope • List of regular polytopes and compounds

corleystideass.blogspot.com

Source: https://en.wikipedia.org/wiki/Pentagon

Share this post